Problem 566
Cake Icing Puzzle
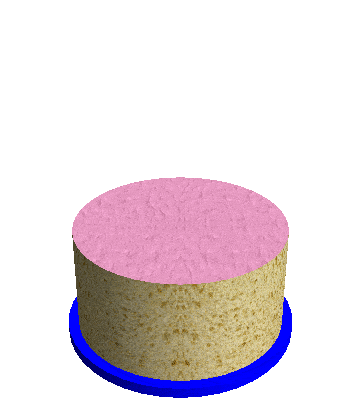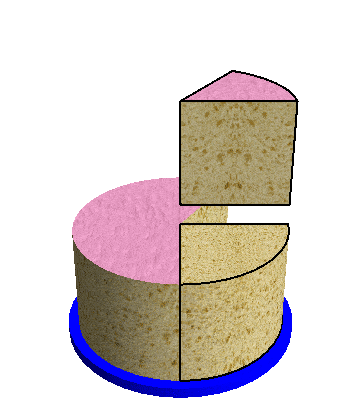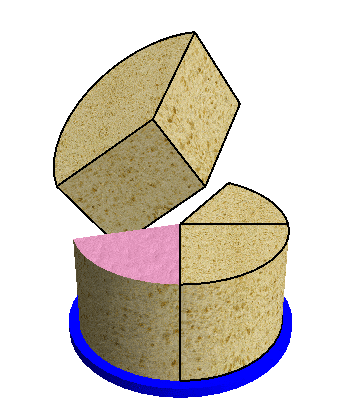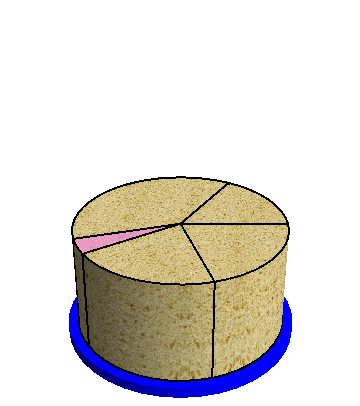
Adam plays the following game with his birthday cake.
He cuts a piece forming a circular sector of 60 degrees and flips the piece upside down, with the icing on the bottom.
He then rotates the cake by 60 degrees counterclockwise, cuts an adjacent 60 degree piece and flips it upside down.
He keeps repeating this, until after a total of twelve steps, all the icing is back on top.
Amazingly, this works for any piece size, even if the cutting angle is an irrational number: all the icing will be back on top after a finite number of steps.
Now, Adam tries something different: he alternates cutting pieces of size $x=\frac{360}{9}$ degrees, $y=\frac{360}{10}$ degrees and $z=\frac{360}{\sqrt{11}}$ degrees. The first piece he cuts has size x and he flips it. The second has size y and he flips it. The third has size z and he flips it. He repeats this with pieces of size x, y and z in that order until all the icing is back on top, and discovers he needs 60 flips altogether.
Let F(a, b, c) be the minimum number of piece flips needed to get all the icing back on top for pieces of size $x=\frac{360}{a}$ degrees, $y=\frac{360}{b}$ degrees and $z=\frac{360}{\sqrt{c}}$ degrees.
Let $G(n) = \sum_{9 \le a < b < c \le n} F(a,b,c)$, for integers a, b and c.
You are given that F(9,10,11)=60, F(10,14,16)=506, F(15,16,17)=785232.
You are also given G(11)=60, G(14)=58020 and G(17)=1269260.
Find G(53).
蛋糕糖衣谜题
亚当在拿他的生日蛋糕玩游戏。
他先切下一块圆心角60度的扇形，把这块颠倒过来，糖衣放在下面。
然后他将蛋糕逆时针转60度，切下相邻的60度扇形，也颠倒过来。
不断重复这一过程，一共重复12次后，所有的糖衣都回到上面了。
令人惊奇的是，对于任意大小的扇形，即使圆心角是一个无理数：经过有限步之后，所有的糖衣都会回到上面。
现在，亚当尝试了一些新花样：他每次交替切割圆心角为$x=\frac{360}{9}$度、$y=\frac{360}{10}$度和$z=\frac{360}{\sqrt{11}}$度的扇形。他先切第一块圆心角为x度的扇形，把这块颠倒过来；再切第二块圆心角为y度的扇形，也颠倒过来；再切第三块圆心角为z度的扇形，也颠倒过来。他按照x，y和z的顺序不断重复这一过程，直到所有糖衣重新回到上面，总共需要60次操作。
如果每次交替切割的圆心角为$x=\frac{360}{a}$度，$y=\frac{360}{b}$度和$z=\frac{360}{\sqrt{c}}$度，记F(a, b, c)为将所有糖衣重新翻回上面所需的最小操作数。
记$G(n) = \sum_{9 \le a < b < c \le n} F(a,b,c)$，其中a，b和c均为整数。
已知F(9,10,11)=60，F(10,14,16)=506，F(15,16,17)=785232。
还已知G(11)=60，G(14)=58020以及G(17)=1269260。
求G(53)。